TEOMAPIN
Solucions a la llacuna entre teoria matemàtica i aplicacions d’enginyeria, dins de l’àrea de sistemes i automàtica, DPI2008-00403.
Objectives
The main objective of this project is to solve practical problems that currently prevent the application of new advanced control techniques to real-world situations of a certain complexity and to generate (and possibly solve) theoretical problems arising from practice.
The issues that need to be addressed during the project are as follows:
SUMMARY
The most innovative technological developments of recent years have been strongly influenced by advances in mathematics and computing. Performance in traditional areas of engineering has significantly increased, and new, more demanding applications have emerged in other fields of engineering and science. There is still a significant gap to be bridged between foundational theory and practical problems. Technical decisions are often made without complete alignment with the latest theory, and the application of an innovative technique relies on these decisions. The issue also arises when the theory does not yet exist or lags behind practice. Usually, this gap is covered through extensive simulations and/or experimental trials, consuming time and resources, e.g., in aerospace applications. Almost no applications perfectly align theoretical models with practice without modifications or adjustments.
This project focuses on the intersection of engineering, computing, and applied mathematics. A deep understanding of practical problems enables us to address various engineering applications. The work area is in systems and automation, particularly in identification, (in)validation, control, implementation analysis, diagnostics, and fault tolerance. The systems to be addressed are general: dynamic models, linear or nonlinear, continuous or discrete time, with bounded uncertainty, parametric or dynamic, time-invariant or time-varying. The gap between theory and practice in this field remains open, and this project aims to solve practical problems that hinder the application of new techniques to real-world situations of certain complexity and to generate (and possibly solve) theoretical issues emerging from practice. The gap will be highlighted, not hidden.
The development is focused on three practical applications that serve as test beds for the obtained results. The SAC group has some experience, experimental accomplishments, and industrial interest in these three applications:
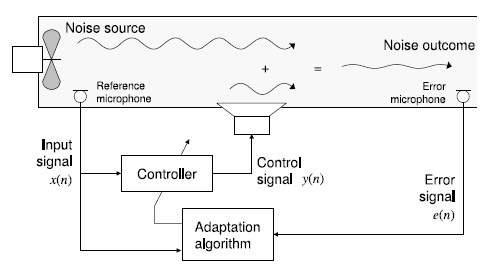
- Active control of acoustic noise (tubes, chambers, helmets)
- Unmanned aerial vehicles (helicopters and planes)
- Wind turbines
Work Carried Out
Optimized determination of the position of sensors and actuators based on maximum achievable performance: This objective was addressed theoretically, and its results were applied to two different Active Noise Control problems, namely the motorcycle and acoustic duct cases. One of the most significant outcomes is a set of measures that allow for comparing the quality of sensor and actuator placement based on practical criteria in performance, robustness, and controller complexity.
Identification of multivariable dynamic systems (MIMO), nonlinear and/or time-varying, represented in continuous time, discrete time, or hybrid combinations. Each application has posed restrictions and highlighted numerous gaps between theory and practice. Specifically, theoretical analysis and practical solutions have addressed identifying plants with variable delays in MIMO systems (irrigation channels), identifying nonlinear dynamics of acoustic noise propagation in various spaces (helmets, ducts, and chambers with windows), and finally, handling identification in UAV-type helicopters, with the complexity of a multivariable, coupled, and unstable system. In this last case, a methodology was developed that allows the application of classical techniques.
Validation (and invalidation) of the above systems against experimental data, considering variables such as external noise bounds (additive) and uncertainty (global or structured, time-varying or invariant, linear dynamic or static nonlinear). A notable contribution in this area is a robust identification/invalidating method for Linear Parameter-Varying (LPV) models. This method complements nominal LPV identification algorithms by adding uncertainty and noise bounds that generate a set of models consistent with experimental evidence.
Numerical issues associated with the use of computers or DSP in the analysis, synthesis, and implementation of controllers. The application of active control and UAV control requires real-time implementation and/or significant computational limitations that prevent the use of arbitrarily large or complex controllers. Additionally, these systems tend to have positive real-part zeros and lightly damped oscillations. Therefore, the design of a low-order controller is a requirement that poses a significant challenge to control theory.
Determining the most suitable type of uncertainty to describe a physical system through a family of models, and computing the least conservative bounds possible. Here, robust and nominal stability and performance have been analyzed in models with uncertain delays, for global dynamic and structured parametric uncertainty structures.
Project funded by:
Most Notable Publications
- R. Sanchez, Y. Bolea, and V. Puig, "MIMO Smith Predictor: General Performance and Robustness results," Journal of Process Control, Vol. 19 (1), pp. 163-177, <doi:10.1016/j.jprocont.2007.12.004>, 2009.
- F. Bianchi and R. Sánchez-Peña, "Robust identification/invalidation in an LPV framework," International Journal of Robust and Nonlinear Control, Vol. 20 (3), pp. 301-312, <doi: 10.1002/rnc.1430>, 2010.
- F. Bianchi and R. Sánchez-Peña, "Interpolation for gain-scheduled control with guarantees," Automatica, Vol. 47 (1), pp. 239-243, <doi: 10.1016/j.automatica.2010.10.028>, 2010.
- R. Castañé-Selga and R. Sánchez-Peña, "A novel design approach for switched LPV controllers," International Journal of Control, Vol. 83 (8), pp. 1710-1717, <doi:10.1080/00207179.2010.490599>, 2010.
Share: